Profitability, one would think, should come quite naturally to a successful, growing SaaS company. But, SaaS startups have consistently struggled to reach profitability. Unprofitable SaaS companies have gone public and remained unprofitable for years after their IPOs, even as they grow revenues into the hundreds of millions of dollars. SaaS companies have eschewed economic convention, with management and investors alike calmly shrugging off the passe’ advice of their obsolete microeconomics professors who cry out in vain from their blackboards that “the role of the firm is to maximize profit.” Many authors have tried to make sense of this morass, two of the more interesting attempts are Bob Warfield’s post entitled Why do SaaS Companies Lose Money Hand Over Fist, and this really cool analysis by Christian Chabot of tech IPOs comparing software companies that were profitable to those that were not profitable at the time of their IPO. And, I’ve certainly made no secret of my own opinion in posts like this one entitled SaaS Failures – The Recurring Revenue Mirage.
This is the third post in a series on SaaS metrics that will unravel the mystery of SaaS profitability (and the lack thereof) from a different angle, a little mathematics. Once and for all we’ll solve the puzzle of why seemingly successful SaaS companies lose money. So, even if you’re not a math junkie like me, please be patient and plough your way through the technical mumbo jumbo. I’ll guarantee some of these results will surprise you!
SaaS profitability is such an important topic that I’m breaking it up into three separate posts (this being the first) that will build on each other by examining three increasingly complex scenarios: 1) stable growth with churn, 2) viral growth, and 3) growth through upselling and upgrades. Along the way, I’ll also introduce a number of new SaaS Metrics Rules-of-Thumb that highlight the impact of customer acquisition costs and recurring cost of service on long term SaaS profitability.
So, let’s get going…
SaaS Metrics Rule-of-Thumb #4
Company Time to Profit Follows Customer Break-Even
This subtle, strikingly simple rule will reverberate through all that comes to follow on SaaS profitability. And, it provides a simple sanity check for predicting when, if ever, your SaaS business will reach profitability. The gist of the rule is that your SaaS recurring revenue over time is nothing more than the sum of its parts. Therefore, the sooner you break even on a single customer, the sooner you will reach profitability as a company.
The chart below visually shows this principle for a SaaS company with a constant rate of customer acquisition eroded by churn which was closely examined in the first post in this SaaS metrics series.
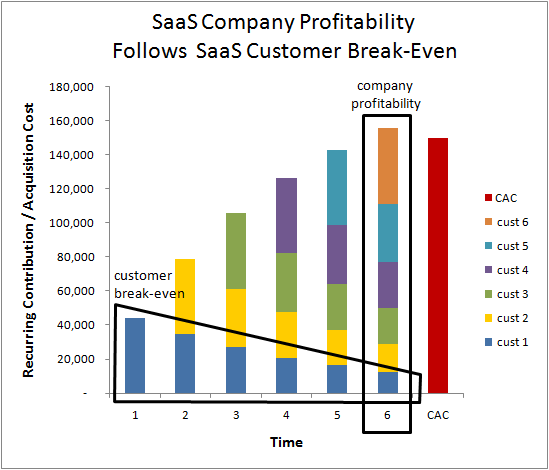
The accumulated recurring contribution of a SaaS company at any time
mirrors the lifetime accumulation of the typical SaaS customer
directly linking company time to profit with customer break-even time.
The average break-even point for an individual customer without churn, “BE0” is given by the following formula:
BE0 = CAC / [ ARR – ACS ]
Where “CAC” is the average acquisition cost per customer, “ARR” is the average recurring revenue per customer, “ACS” is the average recurring cost of service per customer, and the 0 attached to the BE is intended to indicate the absence of churn, i.e., the baseline break-even.
Now let’s compare this simple individual customer SaaS metric with the more complex SaaS metric of company time-to-profit.
At any given time, the rate at which a SaaS company generates profit is given by the following formula:
Profit = P(t) = [ ARR – ACS ] C (t) – CAC x Cnew(t)
Where C is the number of customers and Cnew is the new customer acquisition rate. Time to profit occurs when this expression changes from a negative loss to a positive profit, or when profit is equal to zero.
P(tprofit) = [ ARR – ACS ] C(tprofit) – CAC x Cnew(tprofit) = 0
If we consider the simplest case scenario of a constant new customer acquisition rate with no churn, “b”, then after time t, we will have b x t total customers, giving the following equation for time to profit:
P(tprofit) = [ ARR – ACS ] x btprofit – CAC x b = 0
tprofit = CAC / [ ARR – ACS ] = BE0
In this simplest of cases, time to profit not only follows break-even, it equals break-even. This relationship between company time to profit and break-even time for the single average customer is a completely general principle for SaaS (or any recurring revenue subscription business). Unfortunately, the simple case scenario above is also the best case scenario as spelled out in the next SaaS metrics rule-of-thumb.
SaaS Metrics Rule-of-Thumb #5
Best Case Time to Profit is Simple Break-Even
SaaS Metrics Rule-of-Thumb #2 – New Customer Acquisition Growth Must Outpace Churn states that if you want to break free of the churn limit, then you must increase new customer acquisition to compensate. Unfortunately, this mandate places you neatly between a rock and a hard place with respect to profitability. On the one hand, your customer acquisition costs increase each year, while on the other hand churn is eating away at your ability to break-even on every new customer you bring the door. The net result is that both churn and growth push SaaS profitability out beyond the single customer break-even point in direct relation to their intensity. The higher your churn, the longer it takes to reach profitability. The higher your growth rate, the longer it takes to reach profitability. And, if either your percentage churn rate or growth rate are too high given your customer acquisition cost and recurring contribution, your SaaS business will never reach profitability. The chart below shows this general principle for the simple case of a constant new acquisition rate subject to churn.

For a growing SaaS company subject to churn,
the best case time to profit is the average break-even time for a single customer.
If churn is too high, profitability becomes impossible to achieve.
In the next installment in this series entitled Growing Up Poor – How Foolish SaaS Companies Lose Money, I plan to zero in on the root causes that lead seemingly successful SaaS companies to lose money. And, then move on to the solutions to this obstinate problem. Some of the concepts presented in this post as well as earlier SaaS Metrics Rules-of-Thumb were developed in previous installments in this SaaS Metrics series:
SaaS Metrics Math Notes
SaaS Metrics Rule-of-Thumb #5 – Best Case Time to Profit is Simple Break-Even can be shown using a little math trick. After I show it, I’ll try to explain in plain English to provide a little more insight into its cause (hint: if you don’t like the math, skip to the end). Taking the formula above for time to profit:
P(tprofit) = [ ARR – ACS ] C(tprofit) – CAC x Cnew(tprofit) = 0
and rewriting it in terms of the baseline break-even time, BE0 = CAC / [ ARR – ACS ], gives the following:
C(tprofit) = BE0 x Cnew(tprofit)
Because we are following SaaS Metrics Rule-of-Thumb #2 – New Customer Acquisition Growth Must Outpace Churn, the new customer acquisition rate, Cnew, is always increasing. Since C is just the sum of all the customers acquired at the lower acquisition rates from earlier times, then had we acquired customers at the current acquisition rate the whole time, we would clearly have acquired more customers.
t x Cnew(t) >= C(t)
Substituting this little trick formula into the above gives the following:
tprofit x Cnew(tprofit) >= C(tprofit) = BE0 x Cnew(tprofit)
tprofit >= BE0
What does it mean? In a subscription business, you reach profitability when the contribution from current customers covers the acquisition cost of new customers. This is the essence of SaaS Metric Rule #4 – Company Time to Profit Follows Customer Break-Even. In the base case, everything is constant and customer break-even = company time to profit. But, if you lose customers to churn on the revenue side, while you acquire new customers at a faster rate each year on the cost side, then it takes more current customers to cover your new customers, so the faster you grow, the longer it takes to stack up enough customers to cover your new ones. If you grow too fast, you can never catch up, and you’ll never be profitable…unless you stop growing! Or, you take action to reduce costs. This is the topic of the next installment in the series: Growing Up Poor – How Foolish SaaS Companies Lose Money.
Check out the rest of the SaaS Metrics Rules-of-Thumb
- SaaS Metrics Rule-of-Thumb #1 – SaaS Churn Kills SaaS Company Growth
- SaaS Metrics Rule-of-Thumb #2 – New Customer Acquisition Growth Must Outpace Churn
- SaaS Metrics Rule-of-Thumb #3 – Viral Growth Trumps SaaS Churn
- SaaS Metrics Rule-of-Thumb #4 – Company Time to Profit Follows Customer Break-Even
- SaaS Metrics Rule-of-Thumb #5 – Best Case Time to Profit is Simple Break-Even
- SaaS Metrics Rule-of-Thumb #6 – Growth Creates Pressure to Reduce Total Cost of Service
- SaaS Metrics Rule-of-Thumb #7 – Churn Creates Pressure to Reduce Total Cost of Service
- SaaS Metrics Rule-of-Thumb #8 – Upselling and Upgrades Accelerate SaaS Profitability
- SaaS Metrics Rule-of-Thumb #9 – Joel’s SaaS Magic Number
- SaaS Metrics Rule-of-Thumb #10 – SaaS Customer Lifetime Value Drives SaaS Company Value
Thank you, Joel. This is extremely helpful. However your continuous churn method (great for illustrating concepts) are less practical for real-world SaaS businesses with fixed-period contracts (e.g., monthly, annually). Would I be wrong if I were to say that “the discrete churn approach is more grounded in real-world operations and aligns with how businesses actually calculate and monitor churn”?
Joel – thanks so much for getting back to me. Yes, that does make much more sense. But just wanted to clarify a few things on this.
1) Does this continuous growth rate formula depict a more accurate representation for the real world or is it the standard (100 x 25% = 75) churn formula that does justice? Asking because I can’t find any other resources on examples of churn being calculated via the continuous growth rate.
2) After running both formulas (continuous vs standard), it seems like the continuous function is more lenient on churn where it hits a maximum limit of 400 customers rather than the 300 in the standard. Is this still a fair game for the continuous method?
3) In the future when we try to calculate churn, which method(s) do you recommend I use? Or should I use both?
Thanks again, Joel – really appreciate it!
Hi Shubhang,
I use continuous churn in these analysis, because it makes the math simpler and the answers more elegant.
The main purpose is to illustrate general principles, as opposed to facilitate calculation.
You will ALWAYS use an interval-based churn formula in the real world, i.e., monthly, annual, etc.
Most contracts have fixed periods….and you generally use the fixed period that matches to your contract.
Take a look at this post for the fixed interval point of view.
https://chaotic-flow.com/saas-metrics-faqs-what-is-churn/
You will also see that the math in the post above consists of adding up long series of sums.
Whereas, in this post I am using calculus to quickly get elegant answers…the reason for using continuous churn.
Because this model not only has churn….but it has simultaneous linear growth.
We start with ZERO customers…adding a fixed amount every year….and they are churning at the same time.
Very complicated if you try to use sums….very simple if you use calculus.
However, the two approaches are completely interchangeable mathematically.
One is not more lenient than the other.
Don’t try to convert this model…it is too difficult because of the linear growth aspect.
If there is NO growth…and you just have 100 customers at the beginning of the year…and they churn for a year.
The continuous formula is 100 x ( 1 – e ( – continuous churn x 1 year ))
To convert annual churn to continuous churn, you use the formula: annual churn = 1 – e ( – continuous churn x 1 year )
Your issue is that you are using 25% for both, instead of converting.
25%/year continuous churn implies annual churn of 22.1% = 1 – e(-.25 x 1)
In which case, both approaches yield the exact same result…which they must.
Cheers,
Joel
Got it! Thanks a lot for your responses, Joel. Really appreciate your insight.
Hi Joel, great articles!
I’m just confused about 1 thing that I can’t seem to get over. For calculating the net # of customers after the 25% churn, why did you use the C(t) = bâ„a ( 1 – e-at ) formula instead of just the standard churn formula? In your model, you start with 100 customers and the churn is 25%, which means the net # of customers should be 75 for the first year. In your model using that formula, I’m getting 88 which doesn’t logically make sense. Hopefully, you can clear this up for me! Thanks!
Hi Shubhang,
There are two differences here from the ‘standard’ churn formula:
1) There is a continuous linear growth rate.
New customers are being added at a rate of 100/year,
and simultaneously churning at 25%/year.
2) The 25% churn rate is a continuous churn rate, not an annual churn rate.
So, customers are being added continuously and churning continuously.
To get the equivalent annual churn rate, it is similar to calculating the APR of a loan.
‘Standard’ Annual Churn = ( 1 – e ( -.25) ) = 22.1%
That only gets you to 78% though, to get to 88% the formula is adding 100 users/year simultaneously with churn. We are not starting off year one with 100 users.
We are stating off year one with 0 users….adding at a continuous rate of 100/year.
Without churn, we would add 100 users. But, with it…we lose roughly half of 25% x 100 because we have on average 50 users throughout the year (gross approximation, but should help you make sense of it)
Joel – thanks so much for getting back to me. Yes, that does make much more sense. But just wanted to clarify a few things on this.
1) Does this continuous growth rate formula depict a more accurate representation for the real world or is it the standard (100 x 25% = 75) churn formula that does justice? Asking because I can’t find any other resources on examples of churn being calculated via the continuous growth rate.
2) After running both formulas (continuous vs standard), it seems like the continuous function is more lenient on churn where it hits a maximum limit of 400 customers rather than the 300 in the standard. Is this still a fair game for the continuous method?
3) In the future when we try to calculate churn, which method(s) do you recommend I use? Or should I use both?
Thanks again, Joel – really appreciate it!
Hi,
Very good blog
Please note that time to profit for the 25% churn model isn’t 5.5 years, but 4.82 years.
I calculated like this:
Log BE0*(-churn)+1 / Log 1-churn
Thank you
Hi Yuri
The formula I used is log( 1 – BEo x churn ) / -churn
Which comes from Recurring Operating Profit in Period = Acquisition Cost in Period
Booking Rate x ( ARR – ACS ) x ( 1 – e^(-churn x time) ) / churn = Booking Rate x CAC
( 1 – e^(-churn x time) ) = BEo * churn
time = log( 1 – BEo x churn ) / -churn
Cheers,
Joel
Thanks
But I don’t understand why you use the linear differential formula here…
By you equation with the following details, here is what I get
BE0 = 3
Churn = 0.25
time = Log (1-3*0.25) / -0.25
time = Log 0.25 / -0.25
time = 2.4
Where did I get it wrong?
oh it’s Ln not log
no I see it. but still I can’t understand why exactly to use it like this.
I tried my formula for dozens of variations and it always gave the correct number, which is different that yours here.
If I run it in excel, tab after tab, I get to profit only on the 6th period, not the 5th…
I realize this is years after Joel’s original blog. But reading it in 2018 has given me insight to something that goes back to the 1990s when I helped tech start-ups pitch VCs. When it came to the financial projections, the smart VCs seemed to have only two rules of thumb. One related to sensitivity analysis of the assumptions in the financial projection – something I understood well and was my principal value to start-up clients and partners. The other rule of thumb I accepted but never really understood the VCs’ motivation for it – until I read Joel’s blog on SaaS Rules of Thumb #4 & #5. The ’90s VCs rule of thumb: Don’t go any further if the financial projections don’t demonstrate increasing gross margin (as a %) as sales increase. The value per sale (e.g., LTV – CAC) doesn’t magically increase just because of scale. You have to earn it by “growing profitably” – a lost art in the Unicorn world of the 2010s. As Joel writes: “The gist of the rule is that your SaaS recurring revenue over time is nothing more than the sum of its parts. Therefore, the sooner you break even on a single customer, the sooner you will reach profitability as a company.” Yet again, theoretical (wishful) macroeconomics and practical (hard work) microeconomics follow Yogi Berra: “In theory there is no difference between theory and practice. But in practice there is.”
[…] picture from Joel York gives an even more interesting view how Customer Acquisition Costs (CAC) combined with Churn will […]
[…] that were accumulated to get it in the first place. If you look at the included picture from Joel York (2010), you will see that the ISV will cover the overall customer acquisition costs by signing up new […]
[…] that were accumulated to get it in the first place. If you look at the included picture from Joel York (2010), you will see that the ISV will cover the overall customer acquisition costs by signing up new […]
[…] that were accumulated to get it in the first place. Â If you look at the included picture from Joel York (2010), you will see that the ISV will cover the overall customer acquisition costs by signing up new […]
[…] We have a tendency to find a magic formula for everything and this applies also to SaaS companies. In my research in this topic, I have found a few resources that give some direction of how to evaluate the healthiness of a SaaS business. It is easy to conclude that Monthly Recurring Revenue (MRR) or Average Recurring Revenue (ARR) is the driver for everything and this number is combined with Customer Acquisition Costs (CAC) we will eventually see whether the company will make money or not. If we add Average Recurring Cost per Customer (ACS) we have the main elements to figure out what the break-even point by using following formula: […]
[…] picture from Joel York gives an even more interesting view how Customer Acquisition Costs (CAC) combined with Churn will […]
Joel,
When calcualting CAC are you using the fully loaded cost of Sales and Marketing including sales base salary and commisions and all marketing salaries & expense?
And Total Cost of Service are the expense directly realted to onboarding and supporting that customer going forward?
All these subtractd from Revenue effectivly reveals contribution margin? which is what we have left to pay fixed overhead (G&A, R&D, etc.) and make a profit?
Thanks!
[…] SaaS Metrics Rule-of-Thumb #4 – Company Time to Profit Follows Customer Break-Even […]
[…] and kick starting cash flow as soon as practical is key to success. A good reference on this Joel York’s SaaS Metrics Rule-of-Thumb #4: Company time to profit follows time to break even. You can’t prove your assumptions around […]
Great post. I think people put too much faith in scale economies reducing variable costs such as CAC. If the math doesn’t look good fairly early on, as a VC, I don’t expect it to get dramatically better later (it could happen but I would not count on it).
As companies grow to large scale, I’ve always been amazed by how much they keep spending on marketing/sales. The most easy examples are in consumer businesses where billions get spent on advertising but I think its true in SaaS and B2B in general.
Hi Britta,
Creating economies of scale to lower total cost of service (both CAC and ACS) is a central theme that I plan to drill down on in the next post in the series, and is essential to accelerating profitability.
WRT the model itself, I made a conscious choice to use “average” CAC/ACS values and exclude fixed costs for a number of reasons (fixed relative to increasing customers).
1) The model is meant to facilitate intuition and decision making for SaaS executives in general more than to predict the actual profitability of any single SaaS company. As such, the results should be read like this “If my current revenue/costs/growth/churn is given by ARR/CAC/ACS/g/a, what will happen if I do nothing to improve the situation? Am I OK or am I in trouble?”
2)Taking the approach above dramatically simplifies the math. I found that a more complex model made the math unbearably cryptic without increasing understanding
(See 3b below for the complicated approach)
3) There are a number of ways to include fixed costs in the model
a) Use the approach of 1 above and say, “OK, these results are bad, how can I improve them?” Make a change, recalculate the average values, and run the model again.
b) Make CAC/ACS a decreasing function of the number of customers. Sound more accurate,
but including explicit economies of scale in the math gets really indecipherable without much new knowledge.
c) Treat fixed costs as external, and then the BE0 is not actually breakeven, but the point at which you’ve covered your variable costs…and can now start thinking about covering your fixed costs…so true break even would be longer (again variable/fixed with number of customers)…again, I chose a) to keep the model simple.
4) Lastly….but MOST importantly. There really are not that many economies of scale
for total cost of service outside the basic infrastructure TCO cost reduction,
….unless your work very very hard to make it so The bulk of the costs
in customer acquisition, onboarding, support, etc. scale directly with the number of customers when they are done by people, manual labor. Scale economies require process automation and customer self- service…which is hard work.
So, back to 1) and the approach…what happens if I don’t create economies of scale (I use of the word “create” intentionally to emphasize the point).
See
https://chaotic-flow.com/saas-tco-the-mirror-image-of-total-cost-of-service/
Stay tuned for the next post in the series…the next SaaS metrics rules of thumb basically say, “you best create economies of scale if you want to be profitable any time soon.”
Thanks for the question. Apologies for the lengthy answer.
Cheers,
Joel
Insightful content.
Joel- Great post for anybody running a SaaS business. Still chewing on the point you made about the rate of growth negatively impacting time to profit, but Benny’s comments above clarified some of it. Afterall, isn’t SaaS inherently based on the promise of economies of scale? In other words, wouldn’t you anticipate both your acquisition costs, as well as your service costs to reduce over time and with a growing customer base on a per-customer basis? Certainly, growth will cause increases to your cost structure, when you have to add that extra server, or hire that extra person, but should be absorbed by the customer base provided your growth continues and the contribution per customer covers more than the variable costs per customer, no?
Thanks for putting the math together – extremely helpful.
http://twitter.com/Britta_SF
Joel,
I like this analysis. The formulas you presented make sense (I forgot how much I used to love math 🙂 ), but I think that we have to
1. separate the company “flat” costs (R&D, Overhead, etc) from the CAC, and
2. to consider the fact that in order to be successful a SaaS company’s CAC should decrease dramatically over time. if it does – thats where the profitability should come from, the idea is that CAC should be lower then even a single year’s income from a given customer. if it does not converge to this – then its a giant challenge since you will always need X salesreps/marketing.expenses/etc per Y customers.
Yes, SaaS companies do have to deal with the fact that expenses are frontloaded while income is backloaded. but….
1. the sales cycle for many SaaS companies is getting shorter as SaaS is gaining acceptance
2. marketing is getting cheaper in the age of social media
3. individual companies’ CAC should get shorter if they can properly target their lead generation machines and more importantly – fine tune their sales cycles.
Benny Shaviv.